Maximum White Subtree

- 给定一棵 $n$ 个节点无根树，每个节点 $u$ 有一个颜色 $a_u$，若 $a_u$ 为 $0$ 则 $u$ 是黑点，若 $a_u$ 为 $1$ 则 $u$ 是白点。 - 对于每个节点 $u$，选出一个**包含** $u$ 的连通子图，设子图中白点个数为 $cnt_1$，黑点个数为 $cnt_2$，请最大化 $cnt_1 - cnt_2$。并输出这个值。 - $1 \leq n \leq 2 \times 10^5$，$0 \leq a_u \leq 1$。

You are given a tree consisting of $ n $ vertices. A tree is a connected undirected graph with $ n-1 $ edges. Each vertex $ v $ of this tree has a color assigned to it ( $ a_v = 1 $ if the vertex $ v $ is white and $ 0 $ if the vertex $ v $ is black). You have to solve the following problem for each vertex $ v $ : what is the maximum difference between the number of white and the number of black vertices you can obtain if you choose some subtree of the given tree that contains the vertex $ v $ ? The subtree of the tree is the connected subgraph of the given tree. More formally, if you choose the subtree that contains $ cnt_w $ white vertices and $ cnt_b $ black vertices, you have to maximize $ cnt_w - cnt_b $ .

The first line of the input contains one integer $ n $ ( $ 2 \le n \le 2 \cdot 10^5 $ ) — the number of vertices in the tree. The second line of the input contains $ n $ integers $ a_1, a_2, \dots, a_n $ ( $ 0 \le a_i \le 1 $ ), where $ a_i $ is the color of the $ i $ -th vertex. Each of the next $ n-1 $ lines describes an edge of the tree. Edge $ i $ is denoted by two integers $ u_i $ and $ v_i $ , the labels of vertices it connects $ (1 \le u_i, v_i \le n, u_i \ne v_i $ ). It is guaranteed that the given edges form a tree.

Print $ n $ integers $ res_1, res_2, \dots, res_n $ , where $ res_i $ is the maximum possible difference between the number of white and black vertices in some subtree that contains the vertex $ i $ .

9
0 1 1 1 0 0 0 0 1
1 2
1 3
3 4
3 5
2 6
4 7
6 8
5 9

2 2 2 2 2 1 1 0 2

4
0 0 1 0
1 2
1 3
1 4

0 -1 1 -1

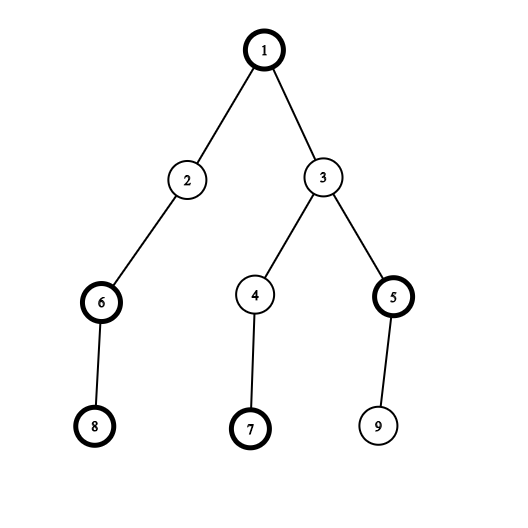
The first example is shown below:  The black vertices have bold borders. In the second example, the best subtree for vertices $ 2, 3 $ and $ 4 $ are vertices $ 2, 3 $ and $ 4 $ correspondingly. And the best subtree for the vertex $ 1 $ is the subtree consisting of vertices $ 1 $ and $ 3 $ .